G.I.A.'s basic premise for the current round diamond cut grading system is that there is no ideal combination of proportions (table percentage, crown angle, pavilion angle, etc.) for round brilliant stones and that many combinations of proportions produce an optimum level of brilliance, fire and scintillation. Preceding the establishment of it's current cut grading system in 2005, G.I.A. published a study in the Fall 2004 edition of Gems & Gemology (a G.I.A. journal), Volume XL. The study is titled "A Foundation for Grading the Overall Cut Quality of Round Brilliant Cut Diamonds." Personally, based partially on G.I.A.'s own studies, personal observation, and discussions with other gemologists, I have concluded that the proportions described in Tolkowsky's American Ideal Cut model developed in 1919 (see below), optimize the play of light in round brilliant diamonds and that other combinations of proportions do not produce the same level of brilliance, fire and scintillation.
TOLKOWSKY'S "AMERICAN IDEAL CUT" PROPORTIONS
Table: 53%
Crown Angle: 34.5 degrees
Pavilion Angle: 40.75
Pavilion Depth: 43.1% (about 40.75 degrees)
Polish/Symmetry: (Unspecified)
Star Length: (Unspecified) *
Lower Girdle Facet Length: (Unspecified)*
Girdle Thickness: (Unspecified)
Culet Size: (Unspecified)
* NOTE: The current G.I.A. cut grading system also
includes measurements for the length of star facets and lower
girdle facets. When Tolkowsky developed the American Ideal Cut, the impact of these measurements on play of light was not known.
In the Fall 2001 edition of Gems & Gemology, Volume XXXVII (a journal of the Gemological Institute of America) published a study titled "Modeling the Appearance of the Round Brilliant Cut Diamond: an Analysis of Fire and More About Brilliance." The three stones referred to in Table 2 (page 179) of the study as RD01,RD10 and RD13 (page 179), which most closely resembled Tolkowsky's American Ideal Cut, were the most optically efficient based on numerical ratings for overall brilliance (i.e., Weighted Light Return or WLR) and fire (Dispersed Colored Light Return or DCLR).
The measurements of the three most optically efficient stones are as follows:
RD01-- WLR: 2.83/DCLR: 3.97; Table: 54%; Crown Angle: 34.3 degrees; Pavilion Angle: 40.6 degrees; Star Facet Length: 53.8%; Lower Girdle Facet Length: 81%; Girdle Thickness: 2.9%; Culet Size: .91%.
RD10-- WLR: 2.81/DCLR: 3.89; Table: 54%; Crown Angle: 34.9 degrees; Pavilion Angle: 40.9 degrees; Star Facet Length: 54.7%; Lower Girdle Facet Length: 76%; Girdle Thickness: 3.0%; Culet Size: .70%.
RD13-- WLR: 2.81/DCLR: 4.01; Table: 52%; Crown Angle: 33.7 degrees; Pavilion Angle: 41.1 degrees; Star Facet Length: 63%; Lower Girdle Facet Length: 80%; Girdle Thickness: 3.3%; Culet Size: 1.11%.
When compared to RD01, RD10 and RD13, all of the stones used in the study with larger table percentages (over 60%), had diminished fire as measured by the DCLR. It should also be pointed out that stones with crown angles of less than 33 degrees and those with crown angles of more than 36.5 degrees showed diminished fire (again, as measured by the DCLR) when compared to those that most closely resembled Tolkowsky's American Ideal Cut model. With regard to the pavilion angle, the study showed that stones with pavilion angles of 40.4-41.2 degrees are generally more optically efficient than those that fall outside of this range (as measured by the WLR and DCLR).
DIMONZ' ROUND DIAMOND CUT CRITERIA VS. G.I.A.'S EXCELLENT CUT GRADE
Dimonz' cut grading system is basically a merging of G.I.A.'s Class I cut criteria (used prior to 2005) and the American Gem Societies 000 Ideal Cut criteria (used prior to 2006), with the addition of criteria pertaining to measurements for the length of star facets and lower
girdle facets (which also have a significant impact on brilliance). Like Dimonz' cut criteria, both systems rated round diamonds according to how closely they resembled Tolkowky's American Ideal Cut and less than 3% less of all round brilliant diamonds meet them. The cut grading criteria for these three systems is described, below.
As noted in a study conducted by Gemworld International and published in the July/August 2014 edition of the GemGuide (a wholesale pricing guide primarily for jewelry appraisers), 67.6% or 2/3 of all round brilliant cut diamonds in a sample of 117,679 stones, received an Excellent cut grade from G.I.A. (Excellent is G.I.A.'s highest rating for cut). The November/December 2014 edition of the GemGuide states "approximately 75% of the round diamonds that go through the G.I.A. lab, receive an Excellent cut grade."
Some round diamonds with a 62% table have an Excellent cut grade from G.I.A. as do some with a 31.5 degree crown angle and others with a 41.8 degree pavilion angle. I would not buy any of these stones.
Only about 2-3% of all round diamonds with G.I.A.'s Excellent cut grade meet Dimonz cut criteria described below:
DIMONZ' ROUND DIAMOND CUT CRITERIA FOR G.I.A. CERTIFIED STONES
Table: 53-58%
Crown Angle: 33.5-35.5 degrees
Pavilion Angle: 40.4-41.2 degrees
Pavilion Depth: N/A
Total Depth Percentage: 58.5-63%
Cut Grade: Excellent
Polish/Symmetry: Very Good to Excellent
Star Length: 50-55%
Lower Girdle Facet Length: 75-80%
Girdle Thickness: Thin; Thin
to Medium; Thin to Slightly Thick; Thin to Thick; Medium; Medium
to Slightly Thick; Slightly thick; Medium to Thick. (with no Very Thin, Extremely Thin, Very Thick
or Extremely Thick measurement at any point on the girdle).
Culet: None, Very Small, Small
Approximate Acceptable Range of Average Girdle Diameters (add the minimum and maximum diameters on the G.I.A. report and divide by two)*:
.50 carat (5.1-5.3 mm)-- Ave. 5.2 mm; .75 carat (5.75-6.0 mm)-- Ave. 5.9 mm; .85 carat (6.05-6.3 mm)-- Ave. 6.2 mm; 1.00 carat--(6.45-6.55 mm)-- Ave. 6.5 mm; 1.25 carat-- (6.85-7.1 mm) Ave. 7.0 mm; 1.50 carat (7.3-7.5 mm) Ave 7.4 mm; 1.75 carat (7.6-7.9 mm) Ave. 7.8 mm; 2.00 carat (8.0-8.3 mm) Ave. 8.2 mm; 8) 2.50 carat (8.7-8.9 mm) Ave. 8.8 mm; 3.00 carat (9.2-9.4 mm) Ave. 9.3 mm.
*If
you have to interpolate between sizes, there is a direct linear
relationship. For example, a diamond weighing 1.13 carats
should have an Average Girdle Diameter of approximately 6.75
mm (i.e., halfway between 6.5 mm for a 1.00 carat and 7.0 mm
for 1.25 carat).
An extremely efficient tool for evaluating the cut of round diamonds (as well as fancy shapes diamonds) is the AGA/NAJA Cut Class Grader, developed by David Atlas (assisted by James Jolliff and Joseph Tenhagen) in the early 1990's. It is still in wide use today by gemologists and jewelry appraisers, especially those affiliated with Accredited Gem Appraisers (A.G.A.) and the National Association of Jewelry Appraisers (N.A.J.A.). In this system, round diamonds are divided into one of eight cut categories: 1A, 1B, 2A, 2B, 3A, 3B, 4A and 4B. All of the values needed to use this system are on the G.I.A. round diamond grading reports-- just plug in the values from the G.I.A. report in the link above and you will receive a rating from 1a (best) to 4b (worst) on each of the factors listed below, including a summary cut grade:
Table Percentage
Crown Angle
Crown Height
Pavilion Depth
Percentage
Girdle Thickness
Total Depth Percentage
Polish
Symmetry
Total Cut Grade
Unless otherwise specified, in addition to meeting the Dimonz cut criteria (above), all of the round diamonds sold by Dimonz meet this system's best cut classification (Class 1a or 1b).
-------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
DISCUSSION
OF "IDEAL CUT" DIAMONDS
The
G.I.A. Class I cut and the pre-2006 A.G.S. 000 Ideal cut grades of a round brilliant diamond were generally based on
how closely a round diamond conformed to the "American Ideal Cut".
The Ideal Cut was developed by a mathematician named Tolkowsky
in 1919 (the Ideal Cut is a successor to European Cut and Mine
Cut diamonds but represented a dramatic improvement in optical
efficiency). The purpose of the Ideal Cut was to produce
the most brilliant diamond possible.
There
are some diamond manufacturers who specialize in fine cut stones
that closely resemble Tolkowsky's model and have excellent cutting
detail. "Super-Ideal" cut diamonds (which cut very closely to
Tolkowsky's original model, and have superior cutting detail)
and "Hearts and Arrows" diamonds ("ideal cuts" which produce
a distinctive hearts and arrows image when viewed through a
special device) are examples, as are diamonds cut by Lazare
Kaplan (a firm specializing in ideal cuts). However, many trademarked ideal cut diamonds are more expensive than similar round brilliants available in the market.
There
are also many variations of Tolkowsky's ideal cut which employ
the same basic faceting arrangement (i.e., 58 facets) and have
similar proportions, such as the "Eppler Fine Cut"; the"Scandinavian
D.N."; and the "Johnson and Rosch" model (note: this model differs
significantly from Tolkowsky's in it's crown and pavilion angles).
In addition, there are specialty cut round stones which generally
have more facets in a different arrangement and are purported
to be more optically efficient than the Tolkowsky's Ideal Cut,
e.g., the "Jubilee Cut" (80 facets), the "King Cut"(86 facets)
, the "Magna Cut"(102 facets), the "Leo" and the "Royal 144" and numerous other variations on different shapes(144 facets).
However, round diamonds with one of these specialty cuts are
generally difficult to find at workable prices and, in my opinion,
may not produce a significant improvement in brilliance over
Tolkowsky's "ideal cut" (although this may depend largely on how light return is measured). Also, there is the matter of liquidity. It is important that diamonds have and retain monetary value and liquidity, even though they may never be sold (e.g., diamonds used in engagement rings). I do not believe that specialty cut diamonds have the same liquidity or intrinsic value in the open market as traditionally cut stones with the 58 facet arrangement of the modern round brilliant.
------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
A.G.S.'s PRE-2006 "000" IDEAL CUT CRITERIA
This
diamond cut grading system was originally developed by Robert
M. Shipley, known as the "Father of Gemology in America." Mr.
Shipley was the founder of both the American Gem Society and
the Gemological Institute of America. Under this system, the
cut grade of a diamond is graded on a scale from 0 (ideal) to
10 (very poor) based on Table %,Crown Angle, Pavilion Depth,
Pavilion Angle, Polish, Symmetry, Girdle Thickness and Culet
size.
Prior to 2006, the American Gem Society (A.G.S.) issued diamond grading certificates which assigned an ideal cut grade (AGS 0 or AGS 000), which was roughly equivalent to G.I.A's Class I cut grade and, like Class I cut stones, comprised about 3% or less of all round diamonds manufactured. The ideal cut criteria for A.G.S. certificates used prior to 2006 is as follows:
Table: 52.4-57.5%
Crown Angle: 33.7-35.8 degrees
Pavilion Angle: 40.15-41.20 degrees
Pavilion Depth: 42.2-43.8%
Polish/Symmetry: Ideal (A.G.S.)-which, in my opinion, is
roughly equivalent to G.I.A.'s Very Good or Excellent
Ave. Girdle Thickness: Thin, Medium or
Slightly Thick
Culet Size: None, Very Small, Small, Medium
--------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
G.I.A.'S CLASS I CUT, USED PRIOR TO 2005:
CLASS
I (the top cut grade, resembling
the "Ideal Cut"):
Table: 53-60%
Crown Angle: 34-35 degrees
Pavilion Angle: (approx. 40.6-40.9 degrees, based on the pavilion depth of 43%)
Pavilion Depth: 43% (rounded to the nearest 1/2%)
Polish/Symmetry: Very Good to Excellent
Girdle Thickness: Medium to
Slightly Thick
You would generally pay approximately 15% more for diamonds
with a Class I cut grade than for round diamond wholesale prices listed in The Guide (published by Gemworld International, Inc., 1997-1998, Volume 16). This volume of The Guide also noted that some trademark cuts that met the Class I cut criteria sold for considerably more than the 15% premium, As previously stated, less than 3% of all round stones met the criteria for G.I.A.'s pre-2005 Class I cut, vs. 67.6%-75% which currently meet G.I.A.'s Excellent cut criteria.
-------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
OTHER
VIEWS OF "IDEAL CUT" DIAMONDS
The International Gemological Institute stipulates
that "Ideal Cut" stones have a 53%-60% table; a 33-36 degree
crown angle; a 42%-44% pavilion depth; a thin to slightly thick
girdle; and a total depth of 58.5%-62.5%. The European Gemological
Laboratory's top cut grade is "Exceptional" and is characterized
by a 54%-57% table; a 34-35 degree crown angle; a 42.5%-43.5%
pavilion depth; and a thin to medium girdle (total depth is
unspecified). The top cut grade recognized by the Central
Gem Laboratory (C.G.L.) of Japan have a 52%-58% table; a
33-35 degree crown angle; a 42%-44% pavilion depth; a thin to
slightly thick girdle; and a total depth of 59.2%-62.4%. The
requirements for assigning an "Excellent" cut grade specified
by the Association of Gemological Laboratories (A.G.L.)
of Japan are identical to those of the CGL with the exception
of allowable table % (C.G.L. allows for 52%-58% vis a vis 53%-58%
for A.G.L.). The top grade recognized by the Association
of Japan Gem Trust has a 53%-60% table; a 33-35 degree crown
angle; a 43% pavilion depth; a thin to slightly thick girdle;
and a total depth of 59%-63%.
--------------------------------------------------------------------------------
--------------------------------------------------------------------------------
THE GENUINE IDEAL CUT DIAMOND-- HOW RARE??
A study
published in the Fall 1998 edition of G.I.A.'s publication,
Gems & Gemology (titled: "Modeling the Appearance of the Round
Brilliant Cut; An Analysis of Brilliance"). it was pointed out that of the 67,621 diamonds
analyzed, only 2,051 or 3% had proportions that fell into the
"ideal" range, which they defined for purposes of this study
as: a 53%-57% table; a 34.0%-34.9% crown angle; and a 42.5%-44%
pavilion depth* which converts to a 40.2-41.2 degree pavilion
angle. How
many of the 3% of diamonds with ideal proportions met the other
criteria for an Ideal Cut diamond, i.e. proper girdle thickness
and Very Good to Excellent on Polish and Symmetry grades? We're
probably talking about 2% of available diamonds.
If
you want to purchase an Ideal Cut diamond, does this mean that
2% of the diamonds in your weight category (e.g. 1 carat, 2
carat, etc.) are worth considering? -- No. In actuality you
may be looking at as few as 1/5th of 1% (one in 500 diamonds
of a particular carat weight)
when you factor in other variables. First of all, you are probably
interested in considering diamonds only in a particular color
and clarity range, e.g., G or H in color and VS1 to SI1 in clarity.
You may also want to eliminate from consideration diamonds that
have medium, strong or very strong fluorescence. Most importantly, you
probably want to eliminate at least 80% of those remaining on
the basis of cost. Remember, because of their scarcity and the
demand for Ideal Cut diamonds, finding a good deal
on one is often very difficult.
WHY AREN'T MORE DIAMONDS CUT WITH DIMONZ' IDEAL PROPORTIONS
The
reason is that diamonds are sold by weight. Consequently, cutters
tend to change the optimum proportions of round diamonds to
retain weight from the rough diamond. The same rough diamond
that produces an "Ideal Cut" 1.30 carat diamond might produce
a poorly cut 1.50 carat diamond by changing the proportions
(e.g., giving the stone a larger table, changing the crown angle,
cutting it too deep, making an extra thick girdle, etc.). The
poorly cut 1.50 carat stone sells for significantly more than the 1.30
carat "Ideal Cut" stone of the same color and clarity. Not only is there a .20 carat gain
in weight, the heavier diamond costs more per carat because
the per carat price increases as diamonds increase in weight.
--------------------------------------------------------------------------------
IMPORTANT
TERMS RELATED TO DIAMOND CUT
In
this section, the terms used in the discussion above, are defined.
Also discussed is how each of the factors, impacts the brilliance
and overall optical efficiency of round brilliant diamonds.
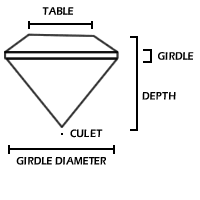
Diagram A
TABLE
PERCENTAGE- This is one of the most important measurements
of the diamond. This is the relative size of the "table" (the
large flat area across the top of the diamond), expressed as
a percentage of the width of the diamond, i.e., the "girdle
diameter" (see Diagram A). For example, if a diamond with a
6.50 millimeter diameter (i.e., the girdle diameter) has a table
measuring 3.90 millimeters across, the table percentage is 60%
(3.9 mm divided by 6.5 mm = 60%). Generally
speaking, the smaller the table, the better the play of light
in the diamond (unless the table is under 52.5%, which is rare).
Dimonz' cut criteria, requires a table percentage of 53%-58%.
Why
are small tables desirable? There are two types of brilliance
which come from a diamond: "life" and "fire" (see diagram B).
The "life" of the diamond is the white light that is reflected
from the table. The "fire" of the diamond is the multicolored
(blue, red, green, orange, yellow and violet), scintillating
light that reflects through the angled part of the crown of
the diamond. The "fire" is regarded as being more beautiful
than the "life" or white light. Stated simply, smaller tables
are desirable because, diamonds with small tables have more
fire and are more aesthetically pleasing than diamonds with
larger tables. However, diamond cutters frequently cut large
tables to save weight from the diamond rough (note: since diamonds
are sold by carat weight, manufacturers generally make more
money this way, although the play of light in the diamond is
negatively affected). You generally pay more for a diamond with
a smaller table because of the greater weight loss from the
rough diamond.
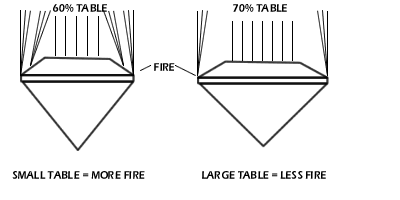
Diagram B
CROWN ANGLE - is the angle formed where the girdle intersects
with the crown (please refer to the diagram on the G.I.A. report, above). This angle
forms a sort of prism that changes the white light reflected
from inside the diamond through the crown into the multicolored "fire." If the crown angle is too shallow or too deep, the fire
of the diamond is diminished. Dimonz' cut criteria, requires a crown angle of 33.5 to 35.5 degrees on the G.I.A. report.
TOTAL DEPTH PERCENTAGE - On a G.I.A. Certificate, this figure
represents the depth of the diamond expressed as a percentage
of it's width. To get the "depth percentage", you take the depth
of the stone in millimeters (the last figure under measurements
on a G.I.A. report) and divide by the diameter (i.e., the Average
Girdle Diameter). Generally speaking,
the Depth Percentage should be between 58.5% and 63% of the width of the stone. Shallow stones with a depth percentage of less than 58% are
called "spready makes" in the gem trade. They appear to be larger
than normally cut stones of the same weight because they are
wider but they tend to be less brilliant because the pavilion
angle is too shallow and light leakage results (Diagram C, far
right figure). Stones with a depth of over 64% are termed "heavy"
or "deep makes" (Diagram C, middle figure). These stones will
be less brilliant than normally cut stones and may appear to
be dark because much of the light leaks out of the bottom or
pavilion of the stone because the pavilion angle is too steep.
Also called "Nailheads" (because they look dark), these stones
also appear to be smaller than normally cut stones of the same
weight because much of the weight is in the depth of the stone.
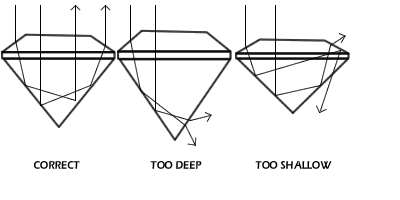
Diagram C
PAVILION
ANGLE -- This
angle, which correlates directly to the pavilion depth, is the
most important measurement in determining the brilliance of
a diamond. If the pavilion angle is too shallow, the diamond
is less brilliant because of light leakage (Diagram C, above,
far right figure). If the pavilion angle is too steep, you also
get light leakage (Diagram C, above, middle figure). Dimonz' cut criteria, requires a pavilion angle of 40.4 to 41.2 degrees on the G.I.A. report.
PAVILION
DEPTH PERCENTAGE -- This measurement is just another way of expressing the pavilion
angle. It is the distance between the girdle and the culet (point
at the very bottom of the diamond) expressed as a percentage
of the girdle diameter of the diamond. For example, if the distance
between the girdle and the culet is 2.8 mm and the diamond has
a girdle diameter of 6.5 mm, the pavilion depth is 43% (2.8
mm divided by 6.5 mm = .43 or 43%). The pavilion depth, like
the pavilion angle, determines how much of the light which enters
the diamond is reflected back to the eye as brilliance. If the
pavilion depth is too shallow or too deep, the diamond is less
brilliant because less light is reflected back to the eye (see
the middle and far right figures in Diagram C, above, i.e.,
either the light leaks out of the back of the stone or at an
angle where it can't be seen).
GIRDLE (see Diagram D, below)- This factor measures the thickness of
the "girdle" or the outside circumference of the diamond. There
are typically two measurements given on a G.I.A. Report. The
first measurement reflects the minimum thickness and the second
reflects the maximum thickness for the girdle of the diamond.
If the girdle is the same thickness all the way round, there
will be only one measurement given. The measurements are as
follows: Extremely Thin, Very Thin, Thin, Medium, Slightly Thick,
Thick, Very thick, Extremely Thick.
Diagram D
Dimonz' cut criteria, allows a wide range of girdle thicknesses-- Thin; Thin
to Medium; Thin to Slightly Thick; Thin to Thick; Medium; Medium
to Slightly Thick; Slightly thick; Medium to Thick. Do
not buy a diamond that has "extremely thin" as one of the girdle
measurements -- it may be prone to chipping along the girdle.
Don't buy a diamond that has " very thick" or "extremely thick" as one of the girdle measurements-- the cutter has added unnecessary
weight to the diamond which you will pay for! Also don't buy
or those with a single measurement of "thick." Again,
the cutter has added unnecessary weight to the diamond.
CULET
(Diagram E) - The culet is a very small facet on the pointed
end of the diamond. Dimonz cut criteria requites any one of the following culet sizes: None, Very Small, or Small. Do not buy a round
diamond with a medium, large or very large culet. They may be eye visible through the top of
the stone, appearing as a dark spot or small black hole in the
center of the diamond.
Diagram E
THE FINISH GRADE FOR A DIAMOND CONSISTS OF TWO ELEMENTS-- POLISH AND SYMMETRY
POLISH- This element measures the quality of the finish
on the surface of the diamond, e.g., the presence of abrasions,
polishing lines and other surface characteristics that were
made when the diamond was cut. There are five grades- i.e.,
Poor, Fair, Good, Very Good, Excellent. Any
round diamond you consider purchasing should have a polish grade of "Very Good" or "Excellent." However,
please be aware that the Polish grade (like Symmetry) is not as important
as proportion characteristics (e.g., table percentage, crown angle
and pavilion depth) in determining the optical efficiency of
a diamond. In G.I.A. Diamond Grading laboratories for aspiring gemologists taught in the 1980's, Polish and Symmetry, when considered together, comprised
only 1/5 of the cut grade of a round diamond-- 4/5 of the cut grade was assigned on the basis of proportions (i.e. table%, crown angle, pavilion depth, girdle thickness).
SYMMETRY-
This element measures the shape and overall cutting detail of
the facets. There are five grades- i.e., Poor, Fair, Good, Very
Good, Excellent. As previously noted,
the symmetry grade in combination with any round diamond you consider
purchasing should have a Symmetry grade of
"Very Good" or "Excellent."
HOW
IMPORTANT ARE EACH OF THESE FACTORS ?
How
important is each of these factors in determining the brilliance
of a diamond? Below, I've done something very unscientific.
how important is each factor on a scale from
0 to 10 in determining the optical efficiency of a diamond?--
0 = not important at all; 1-3 = moderately important; 4-7 =
important; 8-10 = (critical; extremely important).
Table
Percentage.....................8
Crown
Angle.............................8
Pavilion Depth/Angle..............10
Girdle Thickness/Culet/Depth
as described, above...............10
Polish......................................4
Symmetry................................4
--------------------------------------------------------------------------------------------------------------------------------------------------------------
It is impossible to tell whether fancy
shape diamonds (i.e., princess, cushion, oval, emerald, Asscher, radiant, pear shape, marquise,
heart shaped, et al) are well cut
from the G.I.A. report. Fancy shape diamonds
that are poorly shaped may sell at a discount of up to 50% (or more) than well-made diamonds of the same color, clarity and carat weight. As noted in Rapaport Diamond Report, a wholesale pricing guide used world-wide, Poorly made (fancy shaped diamonds) often trade at large discounts while well-made stones may be hard to locate and bring premium prices (September 2015 edition).
In my opinion, the majority of fancy shaped diamonds (90% or more) have
been cut improperly to save weight from the rough (since diamonds
are sold by carat weight, saving weight increases profit). Also, since we don't have a recognized standard to evaluate the cut of fancy shaped diamonds like we do for round diamonds (e.g., Tolkowsky's American Ideal Cut) it is extremely difficult, if not impossible, for someone not engaged in the diamond trade to determine whether or not these stones are properly cut.
For
example, many marquise, pear shapes, and ovals
have pronounced "bow-ties" or dark, extinguished areas that
reduce the brilliance of the stones (usually caused by the stone
being cut too deep or too shallow). Generally speaking, princess cuts tend to be cut too deep, look smaller than they should for their carat weight, have
thin crowns and do not play well with light. Emerald cut diamonds are frequently too squarish or have "bulged pavilions"
(extra weight added by distorting the shape of the bottom
part of the stone), or may have wide or narrow corners.
Some pear shapes look more like a shield than a "tear drop"
(a phenomenon known as high shoulders with a flat head). Many
fancy shapes have improper length-to-width ratios, making them
either too fat or too thin to be aesthetically pleasing. Oval and marquise shaped diamonds may be cut too
wide or fat to retain weight from the rough or they may have "flat
wings" (i.e., the four arcs that form the outline of the stone
are flattened rather than rounded), creating an undesirable
shape. Also, G.I.A. reports for fancy shaped diamonds do not contain the crown height percentage (i.e., the height of the crown of the diamond expressed as a percentage of the width of the stone) which is essential in determining whether the stone will play well with light. These are just a few of the problems you could encounter
when buying a fancy shape.
Below are proportional diagrams taken from G.I.A. reports for a round brilliant cut stone (on the left) and a fancy shape diamond (on the right). Not only do G.I.A. reports not provide an overall cut grade for fancy shape diamonds (as they do for round brilliant cut diamonds), as you see below there is very little information contained in the proportional diagrams for fancy shape diamonds as compared to round brilliants.
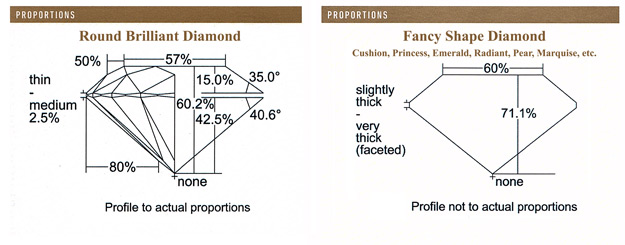
This is one
area where you need the help of an expert who has your interests in mind- not a sales clerk
at a jewelry store or someone who may use his or her knowledge against you. Please call me (Bill Bailey) to discuss
how to evaluate the cut of different shapes and/or find out
what I have in inventory (phone # 703-237-6856). You may also
let me know if you want me to conduct a search for your stone.
GENERAL
RECOMMENDATION: FOR ENGAGEMENT RINGS, BUY DIAMONDS WITH G, H
or I COLOR
Diamonds
are graded on a scale from D (perfectly white) through Z (light
yellow). These letter grades reflect the amount of yellow in
the diamond when the it is viewed through the side, against
a white background , with light that simulates North daylight.
The whiter the diamond (i.e., the less yellow) the more beautiful
and valuable it is. D is perfectly colorless or white and Z
is yellow.
For
an engagement ring, you generally want to select a diamond that
doesn't show any trace of yellow after it is mounted. Round
diamonds from D through I color will appear white when they
are mounted. This begs the question: "If all diamonds graded
'I' or better appear white when mounted, why should I pay more
for a D or E colored diamond than I would for one of I color?"
The
body color of all round diamonds graded D through I appear completely white
when mounted. However, if all else is equal in terms of the
cut of the stones, the whiter diamonds, e.g., the D and E color
stones, will have an aesthetic advantage over those of I color.
Specifically, the brilliance or play of light in the top color
stones may appear somewhat brighter and whiter than those with
a slightly lower color. However, an "ideal" cut H or I colored
diamond that plays beautifully with light, may have a significant
aesthetic advantage over a much more expensive D or E color
diamond that is not as well cut.
I
generally recommend against buying diamonds of D or E color.
If you buy a diamond in this color range, you will pay substantially
more for relatively small differences in appearance. My own
preference is for diamonds of G, H or I in color. G, H and I
color diamonds face up perfectly white when mounted and represent,
in my opinion, the optimum combination of value and aesthetics.
Occasionally, I will carry diamonds of D or E color, but only
when I am able to offer them at an exceptionally good price.
When
mounted, diamonds of J color may show a slight tint but just
as frequently, they look white when they are mounted. Diamonds
of J color need to be evaluated on a case by case basis. Frequently,
diamonds of I or J in color with medium or strong blue fluorescence
will appear one or two colors whiter when mounted than they
actually are. If you want to save money and still have a bright
white looking stone, buying a diamond in this color range with
blue fluorescence is one way to do it.
At
K color and below, diamonds typically show increasing degrees
of yellow, as described below:
The
Gemological Institute of America defines color grades as follows:
| D-F |
Colorless
(appear colorless when mounted) |
| G-J
|
Near
Colorless (G, H and I appear colorless when mounted; J may
be slightly tinted) |
| K-M
|
Faint
Yellow |
| N-R
|
Very
Light Yellow |
| S-Z
|
Light
Yellow* |
| |
*
Diamonds with greater saturation than Z are termed "fancy" colored diamonds. |
Clarity
is the measure of how "pure" the diamond is. Diamonds contain
many types of inclusions. Very small bits of graphite, olivine,
garnet, or other diamond crystals, are common inclusions in
diamonds. Other kinds of inclusions include feathers (small
cracks which can be contained entirely inside the stone or may,
in some cases, break the surface) or "naturals", i.e., slight
indentations on the girdle or outside perimeter of the diamond.
The clarity grade measures how pronounced or observable these
inclusions are.
GENERAL
RECOMMENDATION: BUY A DIAMOND WITH A VS1, VS2 OR SI1 CLARITY
GRADE (Note: As I've noted elsewhere, I only recommend
buying diamonds with G.I.A. Reports).
DIAMOND
CLARITY GRADES
The Gemological Institute of America recognizes the following
clarity grades (in descending order) :
Flawless
- No inclusions when viewed by a trained expert with 10 power
magnification.
VVS1 and VVS2 - "VVS" stands for "very, very slightly
included". Diamonds graded as VVS1 or VVS2 have extremely small
inclusions that are very difficult for a trained expert to find
with the aid of 10 power magnification.
VS1 - "VS" stands for "very slightly included". VS1 diamonds have
inclusions that are very small and discreet and are somewhat
difficult to find with the aid of ten power magnification, even
for someone with training.
VS2
- VS2 diamonds have very small, discreet inclusions that are
somewhat easy to find when examined with 10 power magnification.
Contains no eye visible inclusions.
SI1 - "SI" stands for "slightly included". SI1 stones have small
inclusions that are easy to see with the aid of 10 power magnification
but are totally "eye clean" , i.e., contains no eye visible
inclusions.
SI2 - SI2 stones have inclusions that are very easy to see
with the aid of 10 power magnification but are supposedly* "eye clean",
i.e., contains no eye visible inclusions.
I1 - I stands for "included". I1 stones have inclusions which are
obvious under 10 power magnification and may be eye visible.
I2
and I3 diamonds have large inclusions that are visible to
the naked eye and threaten the beauty and/or durability of the
diamond.
*although some G.I.A. certified SI2 stones have eye visible inclusions.
DISCUSSION:
If
you're looking to save a little money on your diamond, here
is where to do it. With the naked eye, even a trained expert
can't tell the difference between a "Flawless" diamond and a
diamond with a VS1 or VS2 clarity grade, yet a Flawless diamond
may cost two or three times as much as a diamond of the same
color and carat weight . The inclusions in a properly graded
VS1, VS2 diamond are small, even under ten power magnification.
Why pay large sums of money for Flawless or VVS diamonds when
they look the same as VS stones to the naked eye?
Truthfully,
I don't see why anyone would pay one cent more for a Flawless
stone than a VS1 or VS2 stone with a very small, beautiful
crystal that took two to four billion years to form inside the
diamond as it developed a hundred miles deep in the earth's
crust. I actually prefer a VS diamond with a little crystal or
other inclusions that give it a natural character-- not to mention the money you'll save over buying a Flawless or VVS stone of the same quality cut, color and carat weight.
On
the other hand, you want to avoid stones with large or eye visible
inclusions that may affect the beauty and/or durability of the stone,
i.e., those with an SI2, I1, I2 or I3 clarity grade. Some SI1 stones are okay, but to me it is a very broad clarity range-- too broad. Make sure you view any SI1 stone you are considering under at least 10 power magnification with a gemological microscope and do not buy one with any of the following types of inclusions: twinning wisps, laser drill holes, etch channels, knots, chips or cavities.
When
buying "emerald-cut" diamonds, you should request a somewhat
higher clarity grade than you require for a round brilliant. Emerald cut diamonds are a rectangularly
shaped "step cut" stones. Inclusions in emerald cut stones are
visible to the naked eye at higher clarity grades than those
with a "brilliant cut" (e.g., rounds, pears, marquises, hearts,
etc.). If you are buying an emerald cut diamond, I would recommend
a clarity grade of at least VS2 or better.
Another piece of advice-- if you visit a jewelry store, view any stone you are considering under at least 10 power magnification with a gemological microscope. I show diamonds to customers with a StereoZoom Gemscope at up to 60X magnification. Do not use a jeweler's
loupe!-- they are too difficult to use effectively without experience.
After doing this for nearly 30 years, I still use my StereoZoom instead of a jeweler's loupe.
The "per carat" price of diamonds increases significantly as the
carat weight goes up. This is primarily because the rough diamonds
needed to cut larger diamonds is much more scarce than the rough
used to produce smaller diamonds. As you go up in carat weight
the rough used to manufacture diamonds becomes scarcer and scarcer.
Round
Diamonds. The chart below demonstrates the per carat increase
in the per carat price* of one carat round, G color, VS2 clarity and H color, SI1 clarity
diamonds:
| Weight
in Carats |
Price
Per Carat GVS2* |
Price
Per Carat HSI1* |
| .30.-.39 |
$2,400 |
$2,200 |
| .40-.49 |
$2,900 |
$2,500 |
| .50-.69 |
$4,000 |
$3,100 |
| .70-.89 |
$4,800 |
$4,100 |
| .90-.99 |
$6,400 |
$5,500 |
| 1.00-1.49 |
$8,500 |
$7,100 |
| 1.50-1.99 |
$11,500 |
$9,200 |
| 2.00-2.99 |
$15,700 |
$12,000 |
| 3.00-3.99 |
$25,000 |
$15,500 |
Fancy
Shapes (i.e., all shapes other than round) generally sell
for different per carat prices than rounds. This is because
of a number of factors. For some shapes, the rough from which
the diamonds are cut is cheaper than it is for rounds. For some
the weight retention for the rough is greater. Other factors,
such as the amount of labor involved in producing certain cuts
and more irregular demand also affects prices. Below is a comparison
of reasonably well cut, 1.00 carat, G color,VS2 diamonds of various shapes based on wholesale data from the July/August 2014 edition of "The Guide" by Gemworld International (please see below):
| 1.00
carat GVS2 |
%
Difference from Round |
| Round |
0% |
| Pear-Shape/Marquise/Oval/Heart |
-26 |
| Princess Cut |
-33% |
| Emerald
Cut/Radiant |
-45% |
*Prices
quoted in this section are based on the Rapaport Diamond Report,
which reflects the New York high, cash wholesale asking price
for reasonably well cut diamonds. The Rapaport prices are normally
somewhat above actual wholesale prices but below average retail
prices. Rapaport prices are generally above actual wholesale prices
published in "The Guide", a wholesale guide used by appraisers, issued by Gemworld International, Inc.
top

